And speaking of “Apollonian”….
I’ve got that blogger tendency towards rampant free-association. So when I described Jefferson as “Apollonian” in an earlier post today, that started a little drumbeat in my brain which led me to the following—a photo of Balanchine’s ballet “Apollo,” a lovely physical manifestation of Apollonian characteristics:
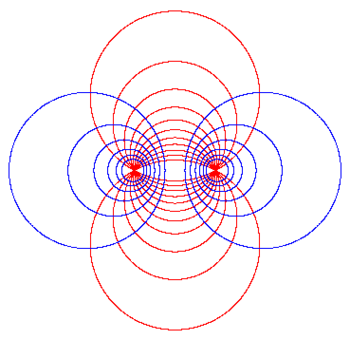
And here are some Apollonian circles. They’re called “Apollonian” after their mathematician founder, Apollonius of Perga. But don’t they show the same qualities?:


Correct me if I’m wrong, Neo, but I sense occasionally (and this is one such occasion) you need to replenish your inner resources spent on analyzing politics by resting your hungry eyes on something unquestionably beautiful – like Balanchine’s ballet or swirl of bookshelves, or your begone garden.
I so understand it.
In fact, I’ll go out immediately for a stroll on the Promenade, to detox myself with thin springerly air, and warmth, and the view of the barges in the Harbor – and the new growth in the park.
Thanks for your posts, last week they were especially good.
Tatyana: You are oh-so-very correct.
I’m about to go outside as well. Wish I could be on the Promenade right about now, though!
And thanks for the compliment!
Hmmm. Is dance where Nietzsche’s Dionysian- Apolonian peronae join hands?
DuMaurier-Smith: Absolutely. I think that’s what attacts me so much about it.
And the act of dancing is so much better than being a mere spectator.
Although being a dance voyeur is rather nice, too :-).
Humm, just where can I get me one of them thar six legged dancers?
Lookin’ mighty nimble.
There are two people and eight legs in that photo.
That just aint right.
I don’t know. Could be a bug’s view of a duck wearing very large sunglasses.
Must…. have…. six-legged…. ballerina… must…
Will…. even…. attend… performance… Swan… Lake…
And for me “Balanchine’s ballet Apollo” is “Stravinsky’s ballet Apollo”
What wonderful music he wrote for it. A kind of transfigured, whitened Tchaikovsky. Sweetness without sugar.
Sheeh! This is about Apollo–and maybe Dionysis. Gods get to have as many legs and arms as they want. True, Shiva has only two legs for his dance, but then he has four arms.
Five hours since asking for critical 6-legged ballerina contact info and still holding.
How long must I refresh page?
A nice compare and contrast is if you take a look at the stuff above and then go to this anti-American piece of art here.
Public places not recommended
Mmmm! Peter Martins in his prime!
And now his son Nilas is dancing the part, too.
Apollonius of Perga came so close to mathematical ideas of modernity, that, if classical tradition could survive only two centuries more, explosive growth of science can begin a thousand years earlier. In fifth century Europe could have nukes, and we would be saved from Islam menace centuries ago.
Vanderleun, be careful what you wish for….are you sure you wan t a contact of a person who can perform kicks x6?
Neo, you’re welcome to join me; the stroll was perfect (but now I don’t want to return to work tomorrow).
[I don’t know if your blog package will allow a third try but here it is. You really need a preview button.]
If it’s orthogonal circles you want, I rather prefer this arrangement, which is fascinating both geometrically and in what it describes. As my late math prof said (between taking a draw on his chalk and trying to write on the blackboard with his cigarette) “a linear fractional transform maps circles and lines into circles and lines [in the complex plane].” Radial lines and circles about the center point map into the two coordinate systems you see.
njcommuter: I used to have a preview button, but it caused a glitch that made it impossible to do links in the comments section at all. The only solution seemed to be to remove it. I would prefer to have a preview function, but I haven’t figured out a way to do a plug-in for one without causing the aforementioned glitch.
My preview button is called metapad. It used to be notepad.
This is called conformal transformation – a mapping conserving angles. A notion from complex analysis. And Apollonius obviously understood it, just as analytical geometry, rediscovered by Rene Descartes two thousand years later.
Indeed and indeed. And electromagnetic theory is one of the foundation applications of complex analysis, hence Mr. Smith and his elegant chart.